
What is the ideal gas law sets the stage for an exploration of a fundamental concept in chemistry and physics. This law, a cornerstone of our understanding of gas behavior, provides a powerful tool for predicting and explaining the properties of gases under various conditions.
The ideal gas law, expressed mathematically as PV = nRT, describes the relationship between the pressure (P), volume (V), temperature (T), and the number of moles (n) of an ideal gas. This simple equation, developed through meticulous experimentation and theoretical analysis, encapsulates the essence of gas behavior and provides a framework for understanding the intricate interactions between gas molecules.
Introduction to the Ideal Gas Law: What Is The Ideal Gas Law
The ideal gas law is a fundamental equation in chemistry and physics that describes the behavior of ideal gases. It establishes a relationship between pressure, volume, temperature, and the amount of gas. This law is widely used in various scientific and engineering applications, such as predicting the behavior of gases in chemical reactions, designing engines, and understanding atmospheric phenomena.
The ideal gas law is based on the concept of an ideal gas, a theoretical model that assumes that gas molecules have no volume and do not interact with each other except through perfectly elastic collisions. While no real gas perfectly fits this model, the ideal gas law provides a good approximation for many gases under certain conditions.
Historical Overview
The development of the ideal gas law was a gradual process involving contributions from several scientists over centuries.
- In the 17th century, Robert Boyle conducted experiments that established the inverse relationship between the pressure and volume of a gas at constant temperature. This relationship is known as Boyle’s Law.
- Later, Jacques Charles and Gay-Lussac independently discovered the relationship between the volume and temperature of a gas at constant pressure. This relationship is known as Charles’s Law or Gay-Lussac’s Law.
- In the 19th century, Amedeo Avogadro proposed that equal volumes of gases at the same temperature and pressure contain the same number of molecules. This concept is known as Avogadro’s Law.
- Finally, in the 1830s, Émile Clapeyron combined the work of Boyle, Charles, and Avogadro to formulate the ideal gas law, which can be expressed as:
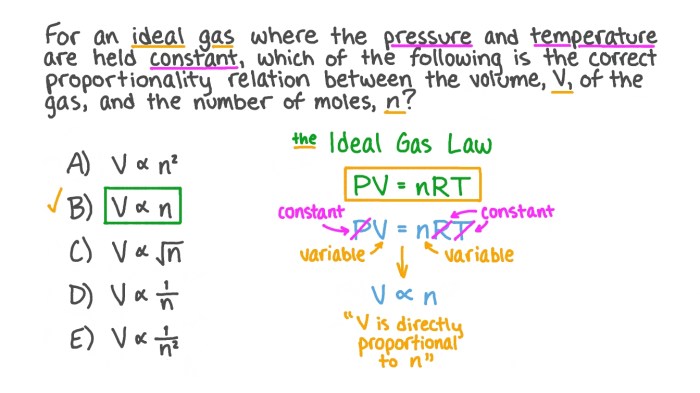
PV = nRT
where P is the pressure, V is the volume, n is the number of moles of gas, R is the ideal gas constant, and T is the temperature in Kelvin.
Assumptions and Limitations
The ideal gas law is based on several assumptions that are not always true for real gases. These assumptions include:
- Gas molecules have no volume. This assumption is valid at low pressures, where the volume occupied by the molecules is negligible compared to the volume of the container.
- Gas molecules do not interact with each other. This assumption is valid at high temperatures, where the kinetic energy of the molecules is high enough to overcome any intermolecular forces.
- Collisions between gas molecules are perfectly elastic. This assumption implies that no energy is lost during collisions, which is not always true in real gases.
These assumptions lead to limitations in the applicability of the ideal gas law. For example, the ideal gas law does not accurately predict the behavior of gases at high pressures or low temperatures, where the volume of the molecules and intermolecular forces become significant. In such cases, more complex equations of state, such as the van der Waals equation, are required.
The Ideal Gas Equation

The ideal gas law is a fundamental equation in chemistry and physics that describes the relationship between the pressure, volume, temperature, and number of moles of an ideal gas. This equation is a powerful tool for understanding the behavior of gases and predicting their properties under different conditions.
The Ideal Gas Equation, What is the ideal gas law
The ideal gas law is expressed mathematically as:
PV = nRT
where:
- P is the pressure of the gas in atmospheres (atm)
- V is the volume of the gas in liters (L)
- n is the number of moles of gas
- R is the ideal gas constant, which has a value of 0.0821 L·atm/mol·K
- T is the temperature of the gas in Kelvin (K)
The ideal gas law states that the product of pressure and volume is directly proportional to the product of the number of moles and temperature. This means that if you increase the pressure of a gas, the volume will decrease proportionally, assuming the temperature and number of moles remain constant. Similarly, if you increase the temperature of a gas, the volume will increase proportionally, assuming the pressure and number of moles remain constant.
Applications of the Ideal Gas Law

The Ideal Gas Law is a fundamental principle in chemistry and physics, providing a powerful tool for understanding and predicting the behavior of gases. Its wide applicability extends across various scientific and engineering fields, offering valuable insights into diverse phenomena.
Calculating Gas Volumes
The Ideal Gas Law allows us to calculate the volume of a gas under specific conditions. For example, in chemical reactions involving gases, we can determine the volume of reactants or products at a given temperature and pressure. Consider the combustion of methane (CH4), a key component of natural gas:
CH4(g) + 2O2(g) → CO2(g) + 2H2O(g)
If we know the amount of methane and the conditions of the reaction, we can use the Ideal Gas Law to calculate the volume of carbon dioxide produced.
Determining Gas Densities
The Ideal Gas Law can be used to determine the density of a gas. Density is defined as mass per unit volume, and the Ideal Gas Law provides a relationship between pressure, volume, temperature, and the number of moles of a gas. By rearranging the Ideal Gas Law, we can obtain an expression for density:
Density = (Molar Mass * Pressure) / (R * Temperature)
Where:
– Molar Mass is the mass of one mole of the gas
– Pressure is the pressure of the gas
– R is the ideal gas constant
– Temperature is the temperature of the gas
This equation allows us to calculate the density of a gas under different conditions, which is crucial for applications such as determining the buoyancy of gases or analyzing the composition of gas mixtures.
Analyzing Chemical Reactions
The Ideal Gas Law plays a significant role in analyzing chemical reactions involving gases. By applying the Ideal Gas Law, we can calculate the volume changes associated with reactions, determine the equilibrium constants for gaseous reactions, and study the kinetics of gas-phase reactions.
For instance, in the Haber process, the synthesis of ammonia (NH3) from nitrogen (N2) and hydrogen (H2) is a crucial industrial process.
N2(g) + 3H2(g) ⇌ 2NH3(g)
Using the Ideal Gas Law, we can calculate the equilibrium constant for this reaction, which helps determine the optimal conditions for maximizing ammonia production.
Table of Applications
| Application | Scenario |
|---|---|
| Calculating Gas Volumes | Determining the volume of a gas produced in a chemical reaction |
| Determining Gas Densities | Analyzing the buoyancy of gases in atmospheric studies |
| Analyzing Chemical Reactions | Studying the kinetics and equilibrium of gas-phase reactions |
| Predicting Gas Behavior | Modeling the behavior of gases in various engineering applications |
| Designing Gas-Related Equipment | Optimizing the design of gas storage tanks and pipelines |
Deviation from Ideal Gas Behavior
The ideal gas law is a useful tool for understanding the behavior of gases, but it is important to remember that it is a simplification. Real gases deviate from ideal behavior under certain conditions. This deviation is due to the fact that real gas molecules have non-zero volume and interact with each other through intermolecular forces.
Factors Contributing to Deviation
The ideal gas law assumes that gas molecules have no volume and do not interact with each other. However, real gas molecules do have volume, and they do interact with each other through intermolecular forces. These forces can be attractive, such as the London dispersion forces, or repulsive, such as the Pauli exclusion principle.
- Intermolecular forces: These forces are attractive forces between molecules, such as hydrogen bonding, dipole-dipole interactions, and London dispersion forces. Attractive forces cause real gas molecules to deviate from ideal behavior by reducing the volume they occupy.
- Molecular size: Real gas molecules have a finite size, unlike ideal gas molecules which are assumed to be point masses. This finite size leads to deviations from ideal behavior at high pressures, where the molecules are closer together and their volume becomes significant compared to the volume of the container.
Examples of Real Gas Behavior
The deviation from ideal behavior is more significant at high pressures and low temperatures. At high pressures, the molecules are closer together, and the intermolecular forces become more significant. At low temperatures, the molecules have less kinetic energy, and the attractive forces between them become more significant.
- Nitrogen: At room temperature and pressure, nitrogen behaves almost ideally. However, at high pressures or low temperatures, it deviates from ideal behavior. This is because the nitrogen molecules are attracted to each other by London dispersion forces.
- Water: Water is a polar molecule, and it has strong hydrogen bonding interactions. This means that water deviates significantly from ideal behavior, even at moderate pressures and temperatures.
The ideal gas law is a good approximation for the behavior of real gases at low pressures and high temperatures, where the intermolecular forces and the molecular size are negligible.
Ideal Gas Law in Chemistry and Physics
The ideal gas law is a fundamental concept in chemistry and physics, providing a framework for understanding the behavior of gases under various conditions. Its applications extend beyond theoretical calculations, playing a crucial role in various scientific disciplines.
Applications in Chemistry
The ideal gas law is indispensable in chemical calculations, particularly in stoichiometry and equilibrium. It enables chemists to predict the volume, pressure, or temperature of a gas involved in a chemical reaction, facilitating the determination of reaction yields and equilibrium constants.
Stoichiometry
Stoichiometry deals with the quantitative relationships between reactants and products in chemical reactions. The ideal gas law allows chemists to relate the volume of a gas to its molar amount, which is essential for determining the amounts of reactants and products involved in a reaction.
For example, consider the combustion of methane (CH4):
CH4(g) + 2O2(g) → CO2(g) + 2H2O(g)
If we know the volume of methane gas at a specific temperature and pressure, we can use the ideal gas law to calculate the moles of methane. This information can then be used to determine the moles of oxygen required for complete combustion and the moles of carbon dioxide and water produced.
Equilibrium
The ideal gas law is also crucial in understanding chemical equilibrium, which describes the state where the rates of the forward and reverse reactions are equal. The ideal gas law helps determine the partial pressures of gases in a reaction mixture, which are essential for calculating the equilibrium constant (Kp).
For example, consider the following reaction:
N2(g) + 3H2(g) ⇌ 2NH3(g)
At equilibrium, the partial pressures of nitrogen, hydrogen, and ammonia can be determined using the ideal gas law. These partial pressures can then be used to calculate Kp, which provides insights into the extent of the reaction at equilibrium.
Applications in Physics
The ideal gas law finds extensive applications in physics, particularly in thermodynamics and fluid mechanics. It helps explain the behavior of gases in various systems, including engines, refrigerators, and atmospheric phenomena.
Thermodynamics
Thermodynamics deals with the relationship between heat, work, and temperature. The ideal gas law is fundamental in understanding the properties of ideal gases, such as internal energy, enthalpy, and entropy. It also forms the basis for deriving other thermodynamic laws, such as the first law of thermodynamics.
For instance, the ideal gas law can be used to calculate the work done by an expanding gas, which is crucial for understanding the operation of heat engines.
Fluid Mechanics
Fluid mechanics deals with the motion of fluids, including gases. The ideal gas law is used to describe the behavior of gases in fluid flow, such as in the study of aerodynamics and meteorology.
For example, the ideal gas law is used to model the behavior of air in aircraft wings, explaining how lift is generated. It is also used in weather forecasting to predict atmospheric pressure and temperature variations.
Summary of Applications
| Discipline | Applications |
|—|—|
| Chemistry | Stoichiometry, Equilibrium |
| Physics | Thermodynamics, Fluid Mechanics |
| Engineering | Design of engines, refrigerators, aircraft |
| Meteorology | Weather forecasting |
| Environmental Science | Air pollution modeling |
End of Discussion
The ideal gas law, despite its simplicity, holds profound implications across various scientific disciplines. It serves as a foundation for understanding chemical reactions, thermodynamic processes, and fluid mechanics. While real gases exhibit deviations from ideal behavior under certain conditions, the ideal gas law remains a powerful tool for approximating gas behavior and providing valuable insights into the world around us.
Q&A
What are some examples of real gases that deviate from ideal behavior?
Real gases deviate from ideal behavior at high pressures and low temperatures due to intermolecular forces and finite molecular size. Examples include carbon dioxide, nitrogen, and oxygen.
How does the ideal gas law relate to the kinetic molecular theory?
The ideal gas law is a macroscopic expression of the kinetic molecular theory, which describes gas behavior at the molecular level. The kinetic molecular theory explains the relationships between pressure, volume, temperature, and the number of moles based on the motion and collisions of gas molecules.




