
What is the newton’s second law of motion – What is Newton’s Second Law of Motion? This fundamental law of physics, discovered by Sir Isaac Newton, governs the motion of objects in our universe. It describes the relationship between force, mass, and acceleration, forming the cornerstone of classical mechanics.
Imagine pushing a grocery cart. The harder you push (force), the faster it accelerates. But what if the cart is full of groceries (increased mass)? It becomes harder to accelerate, illustrating how mass influences motion. This relationship, precisely defined by Newton’s Second Law, governs everything from the flight of a ball to the orbits of planets.
Newton’s Second Law of Motion
Newton’s Second Law of Motion is a fundamental principle in classical mechanics that describes the relationship between an object’s mass, acceleration, and the net force acting upon it. This law forms the cornerstone of our understanding of how objects move and interact with each other in the physical world.
The Fundamental Concept
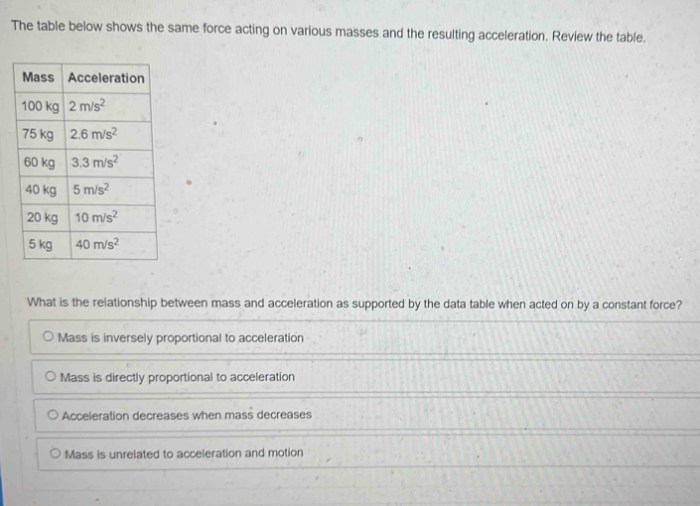
Newton’s Second Law states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. This means that a greater force will produce a greater acceleration, and a more massive object will experience a smaller acceleration for the same force.
Historical Context and Significance
The discovery of Newton’s Second Law of Motion is deeply intertwined with the scientific revolution of the 17th century. Sir Isaac Newton, a renowned English physicist and mathematician, formulated this law in his groundbreaking work “Philosophiæ Naturalis Principia Mathematica” published in 1687. This work revolutionized our understanding of the universe and laid the foundation for modern physics.
Sir Isaac Newton’s Role, What is the newton’s second law of motion
Sir Isaac Newton played a pivotal role in the development of classical mechanics. He was the first to accurately describe the motion of objects using mathematical equations. Newton’s Second Law of Motion, along with his other laws of motion and law of universal gravitation, formed the basis of classical mechanics, a branch of physics that deals with the motion of macroscopic objects. His work provided a framework for understanding the behavior of objects in the physical world, from the motion of planets to the trajectory of a ball thrown in the air.
Statement of the Law: What Is The Newton’s Second Law Of Motion
Newton’s Second Law of Motion is a fundamental principle in physics that describes the relationship between force, mass, and acceleration. It is a cornerstone of classical mechanics and provides a quantitative framework for understanding the motion of objects.
The Mathematical Formula
Newton’s Second Law is mathematically expressed as:
F = ma
where:
- F represents the net force acting on an object, measured in Newtons (N).
- m represents the mass of the object, measured in kilograms (kg).
- a represents the acceleration of the object, measured in meters per second squared (m/s²).
Relationship between Force, Mass, and Acceleration
The formula F = ma reveals the following relationships:
- Direct Proportionality between Force and Acceleration: If the mass of an object remains constant, the acceleration is directly proportional to the net force acting on it. This means that a larger force will produce a larger acceleration, and vice versa. For example, if you push a cart with twice the force, it will accelerate twice as fast.
- Inverse Proportionality between Mass and Acceleration: If the net force acting on an object remains constant, the acceleration is inversely proportional to the mass of the object. This means that a heavier object will accelerate slower than a lighter object under the same force. For example, if you push a heavy box and a lighter box with the same force, the lighter box will accelerate faster.
Force and Acceleration

The concept of force is central to understanding Newton’s Second Law of Motion. Force is essentially a push or pull that can alter an object’s state of motion. This alteration can manifest as a change in the object’s speed, direction, or both.
Types of Forces
Forces play a crucial role in shaping the world around us. Here are some of the most common types of forces:
- Gravitational Force: This is the force of attraction between any two objects with mass. The greater the mass of the objects, the stronger the gravitational force. Earth’s gravity pulls objects towards its center, causing them to fall.
- Frictional Force: This force opposes motion between two surfaces in contact. It arises from microscopic irregularities on the surfaces that interlock and resist sliding. Friction can be helpful, like when it helps us walk or stop a car, or it can be detrimental, like when it slows down machinery.
- Normal Force: This force acts perpendicular to a surface and supports an object resting on it. It is equal in magnitude and opposite in direction to the force exerted by the object on the surface.
- Tension Force: This force arises in a rope, cable, or string when it is pulled taut. It acts along the length of the object and is directed towards the object pulling it.
- Applied Force: This is a force applied directly to an object by an external agent, like a push or a pull.
- Magnetic Force: This force arises from the interaction of magnetic fields. It is responsible for attracting or repelling magnetic objects.
- Electrostatic Force: This force arises from the interaction of electrically charged objects. It can be attractive or repulsive, depending on the charges of the objects.
Relationship Between Force and Acceleration
Newton’s Second Law of Motion states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. This means that:
* A larger net force produces a larger acceleration.
* A smaller mass produces a larger acceleration for the same force.
This relationship can be expressed mathematically as:
F = ma
where:
* F is the net force acting on the object (in Newtons, N)
* m is the mass of the object (in kilograms, kg)
* a is the acceleration of the object (in meters per second squared, m/s²)
For example, if you push a small cart with a force of 10 N, it will accelerate faster than a large cart with the same force applied. Similarly, if you push a cart with a force of 20 N, it will accelerate twice as fast as if you pushed it with a force of 10 N.
Mass and Inertia
Inertia is a fundamental concept in physics that describes an object’s resistance to changes in its motion. It’s a property of matter that dictates how much an object will resist acceleration. The more inertia an object has, the harder it is to get it moving or to stop it once it’s in motion.
Mass as a Measure of Inertia
Mass is a fundamental property of matter that quantifies the amount of matter in an object. It’s directly proportional to an object’s inertia, meaning that the more massive an object is, the more inertia it possesses. This relationship is expressed by Newton’s Second Law of Motion, which states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass.
F = ma
Where:
– F is the net force acting on the object
– m is the mass of the object
– a is the acceleration of the object
This equation highlights the relationship between mass and inertia. A larger mass requires a greater force to achieve the same acceleration as a smaller mass.
Acceleration of Objects with Different Masses
Consider two objects with different masses, subjected to the same force. The object with the smaller mass will experience a greater acceleration than the object with the larger mass. This is because the force applied to both objects is the same, but the smaller mass has less inertia, making it easier to accelerate.
For example, imagine pushing a small car and a large truck with the same force. The car, having a smaller mass, will accelerate more quickly than the truck, which has a larger mass and therefore more inertia.
Applications of Newton’s Second Law
Newton’s Second Law of Motion is a fundamental principle in physics that governs the motion of objects. It states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. This law has wide-ranging applications in various fields, from understanding the motion of celestial bodies to designing everyday objects.
Applications in Physics
Newton’s Second Law finds numerous applications in the realm of physics. It forms the foundation for understanding the motion of objects under the influence of forces. Some notable examples include:
- Motion of Projectiles: The trajectory of a projectile, such as a ball thrown in the air, can be determined using Newton’s Second Law. The force of gravity acts on the projectile, causing it to accelerate downwards. By applying the law, we can calculate the projectile’s velocity, displacement, and time of flight.
- Orbital Mechanics: Newton’s Second Law is crucial in understanding the motion of planets and satellites around stars. The gravitational force between the celestial bodies determines their acceleration and orbital paths. This law enables us to predict the movements of planets, design spacecraft trajectories, and analyze the stability of planetary systems.
- Collisions: When two objects collide, Newton’s Second Law helps us understand the forces involved and the resulting changes in momentum. By analyzing the forces and masses of the colliding objects, we can predict the velocities and directions of the objects after the collision.
Applications in Engineering
Engineers rely heavily on Newton’s Second Law in designing and analyzing various structures and systems. This law allows them to calculate the forces and stresses acting on objects and ensure their stability and safety.
- Structural Design: Civil engineers use Newton’s Second Law to design buildings, bridges, and other structures. They analyze the forces exerted by gravity, wind, and other loads on the structure and ensure that the materials and design can withstand these forces without failure.
- Vehicle Design: Automotive engineers apply Newton’s Second Law to design vehicles. They calculate the forces needed to accelerate and decelerate the vehicle, as well as the forces required for turning and braking. This information helps them optimize the vehicle’s performance and safety.
- Robotics: Robotics engineers use Newton’s Second Law to control the motion of robots. They calculate the forces required to move the robot’s limbs and manipulate objects. This law is essential for designing robots that can perform complex tasks in various environments.
Applications in Everyday Life
Newton’s Second Law is not limited to scientific and engineering applications; it also plays a significant role in our everyday experiences.
- Pushing a Grocery Cart: When you push a grocery cart, you apply a force to it. The cart accelerates in response to the force you apply, and its acceleration is inversely proportional to its mass. A heavier cart will require a greater force to achieve the same acceleration.
- Catching a Ball: When you catch a ball, you apply a force to it to slow it down. The force you apply causes the ball to decelerate, and the deceleration is inversely proportional to the ball’s mass. A heavier ball will require a greater force to stop it.
- Riding a Bicycle: When you ride a bicycle, you apply a force to the pedals to accelerate the bicycle. The force you apply is transmitted to the wheels, causing the bicycle to move forward. The acceleration of the bicycle is proportional to the force you apply and inversely proportional to the combined mass of the bicycle and rider.
Examples of Forces, Masses, and Accelerations
| Scenario | Force (N) | Mass (kg) | Acceleration (m/s²) |
|---|---|---|---|
| Pushing a 10 kg grocery cart with a force of 20 N | 20 | 10 | 2 |
| A 1000 kg car accelerating at 2 m/s² | 2000 | 1000 | 2 |
| A 0.5 kg ball falling under gravity (assuming negligible air resistance) | 4.9 | 0.5 | 9.8 |
Limitations of Newton’s Second Law
Newton’s Second Law of Motion, while a powerful tool for understanding motion in everyday scenarios, has its limitations when applied to extreme conditions or complex systems. These limitations highlight the need for more advanced theories to describe the universe accurately.
Relativistic Speeds
Newton’s Second Law assumes that mass is constant, but this assumption breaks down at speeds approaching the speed of light. According to Einstein’s theory of special relativity, mass increases with velocity, becoming infinite as the speed of light is approached. This means that Newton’s Second Law cannot accurately predict the motion of objects at relativistic speeds.
The relativistic momentum of a particle is given by p = γmv, where γ is the Lorentz factor, m is the rest mass, and v is the velocity.
Quantum Mechanics
At the atomic and subatomic levels, Newton’s Second Law is no longer applicable. Quantum mechanics, which governs the behavior of particles at these scales, introduces the concept of wave-particle duality and the uncertainty principle. These principles imply that the precise position and momentum of a particle cannot be simultaneously determined.
The uncertainty principle states that the product of the uncertainties in position (Δx) and momentum (Δp) of a particle is always greater than or equal to Planck’s constant divided by 4π: ΔxΔp ≥ h/4π.
Non-Inertial Frames of Reference
Newton’s Second Law is formulated for inertial frames of reference, which are frames that are not accelerating. In non-inertial frames, such as a rotating frame, fictitious forces appear that are not caused by physical interactions. These forces, like the centrifugal force and Coriolis force, must be accounted for when applying Newton’s Second Law in such frames.
In a rotating frame, the Coriolis force acts perpendicular to the velocity of an object, causing it to deviate from a straight path.
Conclusion
Newton’s Second Law of Motion is a fundamental principle in physics that governs the motion of objects. It establishes a direct relationship between force, mass, and acceleration. Understanding this law allows us to predict and explain the motion of objects in our universe.
Key Concepts and Applications
Newton’s Second Law states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. This can be expressed mathematically as:
F = ma
Where:
* F represents the net force acting on the object.
* m represents the mass of the object.
* a represents the acceleration of the object.
This law has numerous applications in various fields, including:
* Engineering: Designing structures, machines, and vehicles.
* Space exploration: Calculating the trajectories of spacecraft and satellites.
* Sports: Analyzing the motion of athletes and equipment.
* Everyday life: Understanding the motion of objects we encounter daily.
Significance of Newton’s Second Law
Newton’s Second Law of Motion is crucial for understanding and predicting the motion of objects in our universe. It provides a framework for analyzing and solving problems related to motion, from simple everyday scenarios to complex scientific inquiries. The law has revolutionized our understanding of the physical world and paved the way for numerous technological advancements.
Ultimate Conclusion
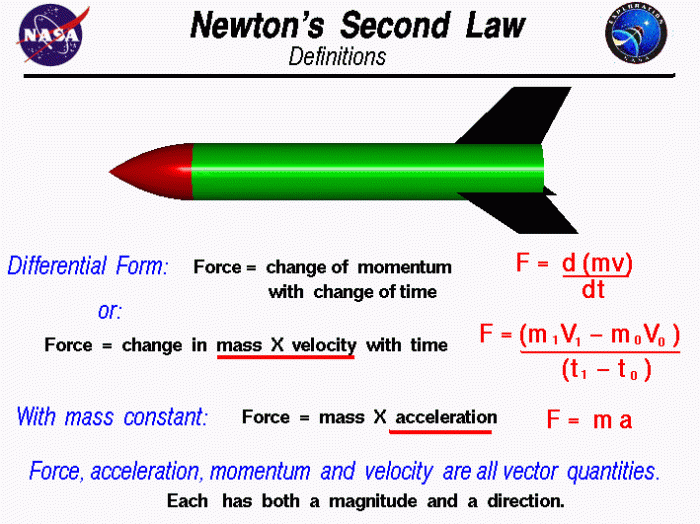
Newton’s Second Law of Motion, expressed by the simple equation F = ma, is a powerful tool for understanding and predicting the motion of objects. It has revolutionized our understanding of the universe, enabling us to design structures, vehicles, and even predict the paths of celestial bodies. While it has limitations in extreme conditions, its core principles remain essential for comprehending the world around us.
Questions and Answers
What is the difference between force and acceleration?
Force is a push or pull that can change an object’s motion, while acceleration is the rate at which an object’s velocity changes.
Can Newton’s Second Law be used to explain the motion of light?
No, Newton’s Second Law is a classical mechanics principle and doesn’t apply to light, which travels at the speed of light and exhibits wave-particle duality, governed by quantum mechanics.
What are some examples of forces in everyday life?
Examples include gravity pulling you down, friction resisting your movement, and the force you exert when pushing a door open.





